TWISTOR DIAGRAMSRecent papers by Andrew HodgesTwistor diagrams home pageSPIRES page Wikipedia page |
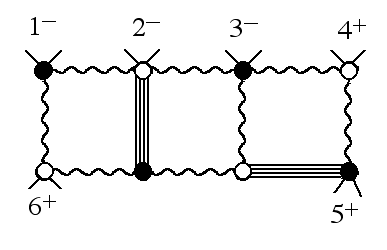
 A twistor diagram for the interaction of three positive-helicity and three negative-helicity gluons. This diagram from my first 2005 paper was the crucial element in the new theory. |
The possibility of a twistor-string connection had been raised long before 2003. In fact in a talk of September 1988, I showed twistor-diagrammatic results on gauge-field scattering with connections to string theory. This was published in The interface of mathematics and particle physics, eds. D. G. Quillen, G. B. Segal and Tsou S. T. (Clarendon Press, 1990). Here is a .pdf file of this paper.
These ideas were pursued throughout the 1990s, with the collaboration of several graduate students. The results of this work will eventually all be useful, but what we missed in that period was the 1986 work of Parke and Taylor which showed the amazing simplifications to be found in pure gauge field scattering amplitudes.
In 2004 I caught up with what Ed Witten had explained about these simplifications, and I could see that the twistor diagram structures that we had studied in the 1980s and 1990s should be highly relevant to the new twistor-based theory of gauge-theoretic scattering. But Witten had not used our twistor diagrams in his paper, and most people at the time would have seen his twistor-string model as something completely different from what had been attempted with twistor diagrams. I gave a presentation at the Oxford conference on strings and twistors, January 2005, suggesting some connections. It ended with a conjecture which was soon to be completely superseded by a new observation.
This is because during February 2005 I saw how I could use the recursion relation recently discovered by Ruth Britto, Freddy Cachazo and Bo Feng. By translating it into twistor diagrams, which turned out to be completely natural, I could represent all the tree-level amplitudes of gauge theory as twistor diagrams. The first paper on this development was Twistor diagram recursion for all gauge-theoretic tree amplitudes, 7 March 2005, at http://arXiv.org/abs/hep-th/0503060.
Results of the 'quadruple cut' analysis of one-loop diagrams (which was also first worked out by the BCF group) are also naturally expressed in twistor diagrams. Some of this material appeared in the talk I gave at the Queen Mary workshop, London University, 3 November 2005. You can download a pdf file of the presentation I gave by going to this site.
At the time these ideas attracted little attention, firstly because the diagram formalism was so completely unfamiliar, and secondly because it went against the grain of current thinking, according to which the new BCF(W) relations were not believed to have anything to do with twistor geometry.
At the end of 2005, I saw a more important new line of development, which positively extended the BCF recursion relation by using super-symmetric twistors, thus making the formalism manifestly super-conformal-invariant. From a computational point of view this effects a great simplification by bringing all the various helicity patterns into one formula. The first paper on this development, Twistor diagrams for all tree amplitudes in gauge theory: a helicity-independent formalism, 29 December 2005, is at http://arxiv.org/abs/hep-th/0512336.
A further paper appeared on 13 March 2006, Scattering amplitudes for eight gauge fields, showing the computational advantage of this new formalism by working out all the 8-field helicity-conserved amplitudes. This is at http://arxiv.org/abs/hep-th/0603101.
I spoke about this work at the workshop on "Twistors, Strings, Gauge Theory and Gravity" organized by Freddy Cachazo at the Perimeter Institute, Waterloo, Ontario, 10-13 September 2006.
I gave a review talk at the London Mathematical Society Durham Symposium on Twistors, Strings and Scattering Amplitudes, 19-26 August 2007. My presentation is available on that site, along with all the other talks, or you can download it here.
In December 2008 I was invited to another Workshop organized by Freddy Cachazo at the Perimeter Institute. One particular reason for holding this workshop was that Freddy Cachazo had been studying my diagram calculus with Nima Arkani-Hamed and his colleagues, and my work had assisted them in arriving at some new and very interesting results. The S-matrix in Twistor Space, by Nima Arkani-Hamed, Freddy Cachazo, Clifford Cheung, and Jared Kaplan, appeared in March 2009 and is available on arXiv here. This introduced the Grassmannian formalism and their new unifying formula for tree-level amplitudes. This work has effectively absorbed and considerably extended the use I had made of twistor diagrams for finding momentum-space expressions for scattering amplitudes.
After the International Workshop on gauge and string amplitudes, at Durham University, UK, 30 March - 2 April 2009, I saw that the new geometrical framework of 'dual conformal invariance' could also be best represented with twistor coordinates, and that this would make it possible to eliminate the 'spurious poles' which have plagued the description of gauge-theoretic amplitudes. A new paper, Eliminating spurious poles from gauge theoretic amplitudes, appeared on 11 May 2009. It is at http://arxiv.org/abs/0905.1473., and has subsequently been published in JHEP, DOI 10.1007/JHEP05(2013)135. I coined the term 'momentum twistors' for the new co-ordinates, and this term has now been widely adopted. A subsequent paper by my colleagues Lionel Mason and David Skinner, Dual superconformal invariance, momentum twistors and Grassmannians, on ArXiv here, extended momentum twistors systematically to super-geometry. The September 2009 paper by Nima Arkani-Hamed, Freddy Cachazo, and Clifford Cheung, The Grassmannian Origin of Dual Superconformal Invariance, on Arxiv here, rapidly showed how momentum (super-)twistors arise naturally in the Grassmannian formalism.
It is fair to say that the momentum twistor formalism is now not only a advance in the theoretical understanding of amplitudes, but has become the most efficient practical method for computing tree-amplitudes for QCD. The computer program of Jacob Bourjaily, explained in his paper of November 2010, Efficient tree-amplitudes on N=4: automatic BCFW recursion in Mathematica, on Arxiv here, makes this claim explicit.
Since 2009, momentum-twistor space has become the focus of new efforts to simplify the analysis of loop amplitudes in supersymmetric gauge theory. I participated in a workshop on Hidden structures in field theory amplitudes at the Niels Bohr Institute, Copenhagen, 12-14 August 2009. A pdf file of my presentation is available on that site, along with all the others. This anticipated the results I published in a new paper on 20 April 2010, The box integrals in momentum-twistor space, at http://arxiv.org/abs/1004.3323, subsequently appearing in JHEP, 10.1007/JHEP08(2013)05. On the same day, my colleagues Lionel Mason and David Skinner published a complementary paper, Amplitudes at weak coupling as polytopes in AdS5, at http://arxiv.org/abs/1004.3498. I gave a talk on this work at the meeting at Queen Mary, University of London, May 2010.
From April to June 2010, I made an extended visit to the Institute for Advanced Study, Princeton, N.J. I also participated in the meeting on scattering amplitudes at the Perimeter Institute, 15-16 September 2010.
This collaborative work led to a A note on polytopes for scattering amplitudes co-authored with Nima Arkani-Hamed, Jacob Bourjaily, Freddy Cachazo and Jaroslav Trnka, 29 December 2010, at http://arxiv.org/abs/1012.6030. (JHEP 1204 (2012) 081). The other authors also published, on the same day, another paper Local integrals for planar scattering amplitudes, at http://arxiv.org/abs/1012.6032. This shows the enormous advances made by this group in finding simple formulas for higher loop amplitudes in momentum-twistor form.
I made brief visits to Princeton and the Perimeter Institute in April and May 2012, combining these with giving talks as part of the Alan Turing centenary year. From 26 to 31 August I participated in a major workshop on amplitudes at the Banff International Research Station, Alberta, Canada, speaking about the gravitational MHV formula. Video of my talk.
A major paper by Nima Arkani-Hamed and five collaborators, Scattering Amplitudes and the Positive Grassmannian, appeared at the very end of 2012. The very beautiful discoveries detailed in this paper have restored the centrality of the twistor diagram formalism, and opened completely new lines of development.
This invited article by me in Nature Physics 9, 205-206, 2 April 2013, doi:10.1038/nphys2597 described the impact of these recent advances on fundamental physics.
The papers on gravitational scattering, as published in 2011 and 2012, were merged and published in July 2013 in the Journal of High Energy Physics, as New expressions for gravitational scattering amplitudes, JHEP 2013:75, doi 10.1007/JHEP07(2013)075.
Thanks to generous invitations, in 2013 I was able to visit the IAS again in March/April. I participated in a CERN workshop in July, in the Clay Mathematics Institute workshop on Number Theory and Physics at Oxford on 2 October, and in a similar meeting at the Simons Center for Geometry and Physics, Stony Brook, NY, in December.I was co-author, with Nima Arkani-Hamed and Jaroslav Trnka, of a further paper: Positive amplitudes in the amplituhedron.